 |
 |
 |
 | Modeling 3-D anisotropic fractal media |  |
![[pdf]](icons/pdf.png) |
Next: von Karman correlation functions
Up: RANDOM FIELDS
Previous: RANDOM FIELDS
I restricted this research to the study of second-order statistics of random
fields. This means the study of random media characterized by Gaussian
distributions, where a Gaussian process is completely specified by its first-
and second-order moments. Furthermore if I define the field 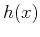 to be
a zero mean process:
to be
a zero mean process:
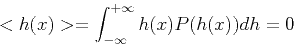 |
(2) |
then 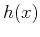 is fully described by its two-point moment that is its autocovariance
function which we write as a function of the correlation function:
is fully described by its two-point moment that is its autocovariance
function which we write as a function of the correlation function:
![\begin{displaymath}
C_{hh}(r)=E[h(x)h(x+r)]=H^2\rho_{hh}(r) \
\end{displaymath}](img17.png) |
(3) |
where 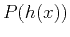 is the probability density function of
is the probability density function of 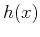 ,
,
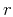 is the lag vector,
is the lag vector, 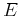 is the expected value,
is the expected value, 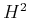 is the variance
(i.e.
is the variance
(i.e. 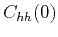 ) and
) and 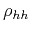 is the three-dimensional correlation function.
Equation (3) shows that the random medium can
be adequately specified by its
autocorrelation function. More generally, an anisotropic random field can be
described by a monotonically decaying autocorrelation function whose rate of
decay depends on direction. The roughness of the medium is function of the
decay rate of the correlation. The Fourier transform of the autocorrelation is
the power spectrum of the field (Bracewell, 1978). Three types of correlation
functions were commonly used in the field of seismic modeling: the Gaussian,
exponential and von Karman functions. These special functions are described by
analytic expressions of their autocorrelations and Fourier transforms.
is the three-dimensional correlation function.
Equation (3) shows that the random medium can
be adequately specified by its
autocorrelation function. More generally, an anisotropic random field can be
described by a monotonically decaying autocorrelation function whose rate of
decay depends on direction. The roughness of the medium is function of the
decay rate of the correlation. The Fourier transform of the autocorrelation is
the power spectrum of the field (Bracewell, 1978). Three types of correlation
functions were commonly used in the field of seismic modeling: the Gaussian,
exponential and von Karman functions. These special functions are described by
analytic expressions of their autocorrelations and Fourier transforms.
Two-dimensional cases have been studied for some
time(Wu and Aki, 1985; Holliger et al., 1993; Goff and Jordan, 1988; Frankel and Clayton, 1986; Holliger and Levander, 1992).
Within the last several years, computer capacity and speed have grown rapidly.
It is now feasible to extend our models and simulations to the
three-dimensional case.
 |
 |
 |
 | Modeling 3-D anisotropic fractal media |  |
![[pdf]](icons/pdf.png) |
Next: von Karman correlation functions
Up: RANDOM FIELDS
Previous: RANDOM FIELDS
2013-03-03
![]() to be
a zero mean process:
to be
a zero mean process: