Next: Constant offset migration
Up: PRESTACK MIGRATION
Previous: Cheops' pyramid
Denoting the horizontal coordinate  of the scattering point by
of the scattering point by 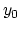 Equation (8.1) in
Equation (8.1) in 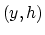 -space is
-space is
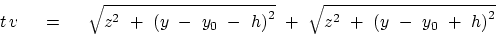 |
(5) |
A basic insight into equation (8.1) is to notice
that at constant-offset 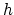 and constant travel time
and constant travel time 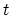 the locus of possible reflectors is
an ellipse in the
the locus of possible reflectors is
an ellipse in the 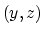 -plane centered at
-plane centered at 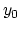 .
The reason it is an ellipse
follows from the geometric definition of an ellipse.
To draw an ellipse,
place a nail or tack into
.
The reason it is an ellipse
follows from the geometric definition of an ellipse.
To draw an ellipse,
place a nail or tack into 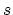 on Figure 8.1
and another into
on Figure 8.1
and another into 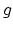 .
Connect the tacks by a string
that is exactly long enough to go through
.
Connect the tacks by a string
that is exactly long enough to go through 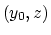 .
An ellipse going through
.
An ellipse going through 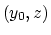 may be constructed
by sliding a pencil along the string,
keeping the string tight.
The string keeps the total distance
may be constructed
by sliding a pencil along the string,
keeping the string tight.
The string keeps the total distance 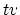 constant as is shown in
Figure 8.3
constant as is shown in
Figure 8.3
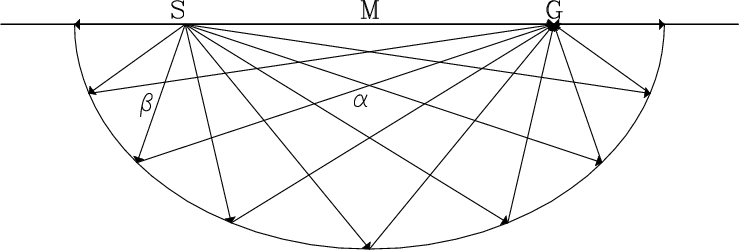
ellipse1
Figure 3.
Prestack migration ellipse, the locus of all scatterers with
constant traveltime for source S and receiver G.
|
|
![[pdf]](icons/pdf.png) ![[png]](icons/viewmag.png) ![[scons]](icons/configure.png)
|
|---|
Replacing depth 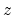 in equation (8.5)
by the vertical traveltime depth
in equation (8.5)
by the vertical traveltime depth
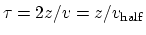 we get
we get
![\begin{displaymath}
t \eq {1 \over 2}\
\left(
\sqrt { \tau^2 + [( y-y_0)-h]^...
...t { \tau^2 + [( y-y_0)+h]^2 / v_{\rm half}^2 }
\
\right)
\end{displaymath}](img32.png) |
(6) |
Next: Constant offset migration
Up: PRESTACK MIGRATION
Previous: Cheops' pyramid
2009-03-16

![]() in equation (8.5)
by the vertical traveltime depth
in equation (8.5)
by the vertical traveltime depth
![]() we get
we get