CWP -- TABLE OF CONTENTS

CWP -- TABLE OF CONTENTS

2001

Nonhyperbolic reflection moveout of 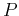$P$-waves: An overview and comparison of reasons [pdf 376K]
Sergey Fomel and Vladimir Grechka
The familiar hyperbolic approximation of $P$-wave reflection moveout is exact for homogeneous isotropic or elliptically anisotropic media above a planar reflector. Any realistic combination of heterogeneity, reflector curvature, and nonelliptic anisotropy will cause departures from hyperbolic moveout at large offsets. Here, we analyze the similarities and differences in the influence of those three factors on $P$-wave reflection traveltimes. Using the weak-anisotropy approximation for velocities in transversely isotropic media with a vertical symmetry axis (VTI model), we show that although the nonhyperbolic moveout due to both vertical heterogeneity and reflector curvature can be interpreted in terms of effective anisotropy, such anisotropy is inherently different from that of a generic homogeneous VTI model.

2006

Time-shift imaging condition in seismic migration [pdf 2.2M]
Paul Sava and Sergey Fomel
Seismic imaging based on single-scattering approximation is based on analysis of the match between the source and receiver wavefields at every image location. Wavefields at depth are functions of space and time and are reconstructed from surface data either by integral methods (Kirchhoff migration) or by differential methods (reverse-time or wavefield extrapolation migration). Different methods can be used to analyze wavefield matching, of which cross-correlation is a popular option. Implementation of a simple imaging condition requires time cross-correlation of source and receiver wavefields, followed by extraction of the zero time lag. A generalized imaging condition operates by cross-correlation in both space and time, followed by image extraction at zero time lag. Images at different spatial cross-correlation lags are indicators of imaging accuracy and are also used for image angle-decomposition. In this paper, we introduce an alternative prestack imaging condition in which we preserve multiple lags of the time cross-correlation. Prestack images are described as functions of time-shifts as opposed to space-shifts between source and receiver wavefields. This imaging condition is applicable to migration by Kirchhoff, wavefield extrapolation or reverse-time techniques. The transformation allows construction of common-image gathers presented as function of either time-shift or reflection angle at every location in space. Inaccurate migration velocity is revealed by angle-domain common-image gathers with non-flat events. Computational experiments using a synthetic dataset from a complex salt model demonstrate the main features of the method.
Imaging overturning reflections by Riemannian Wavefield Extrapolation [pdf 2.1M]
Paul Sava
Correctly propagating waves from overhanging reflectors is crucial for imaging in complex geology. This type of reflections are difficult or impossible to use in imaging using one-way downward continuation, because they violate an intrinsic assumption of this imaging method, i.e. vertical upward propagation of reflection data. Riemannian wavefield extrapolation is one of the techniques developed to address the limitations of one-way wavefield extrapolation in Cartesian coordinates. This method generalizes one-way wavefield extrapolation to general Riemannian coordinate system. Such coordinate systems can be constructed in different ways, one possibility being construction using ray tracing in a smooth velocity model from a starting plane in the imaged volume. This approach incorporates partially the propagation path into the coordinate system and leaves the balance for the one-way wavefield extrapolation operator. Thus, wavefield extrapolation follows overturning wave paths and extrapolated waves using low-order operators, which makes the extrapolation operation fast and robust.

2007

Stereographic imaging condition for wave-equation migration [pdf 840K]
Paul Sava
Imaging under the single-scattering approximation consists of two steps: wavefield reconstruction of source and receiver wavefields from simulated and recorded data, respectively, and imaging from the extrapolated wavefields of the locations where reflectors occur. Conventionally, the imaging condition indicates the presence of reflectors when propagation times of reflections in the source and receiver wavefields match. The main drawback of conventional cross-correlation imaging condition is that it ignores the local spatial coherence of reflection events and relies only on their propagation time. This leads to interference between unrelated events that occur at the same time. Sources of cross-talk include seismic events corresponding to different seismic experiments, or different propagation paths, or different types of reflections (primary or multiple) or different wave modes (P or S). An alternative imaging condition operates on the same extrapolated wavefields, but cross-correlation takes place in a higher-dimensional domain where seismic events are separated based on their local space-time slope. Events are matched based on two parameters (time and local slope), thus justifying the name ``stereographic'' for this imaging condition. Stereographic imaging attenuates wavefield cross-talk and reduces imaging artifacts compared with conventional imaging. Applications of the stereographic imaging condition include simultaneous imaging of multiple seismic experiments, multiple attenuation in the imaging condition, and attenuation of cross-talk between multiple wavefield branches or between multiple wave modes.

2008

Numeric implementation of wave-equation migration velocity analysis operators [pdf 916K]
Paul Sava and Ioan Vlad
Wave-equation migration velocity analysis (MVA) is a technique similar to wave-equation tomography because it is designed to update velocity models using information derived from full seismic wavefields. On the other hand, wave-equation MVA is similar to conventional, traveltime-based MVA because it derives the information used for model updates from properties of migrated images, e.g. focusing and moveout. The main motivation for using wave-equation MVA is derived from its consistency with the corresponding wave-equation migration, which makes this technique robust and capable of handling multipathing characterizing media with large and sharp velocity contrasts. The wave-equation MVA operators are constructed using linearizations of conventional wavefield extrapolation operators, assuming small perturbations relative to the background velocity model. Similarly to typical wavefield extrapolation operators, the wave-equation MVA operators can be implemented in the mixed space-wavenumber domain using approximations of different orders of accuracy. As for wave-equation migration, wave-equation MVA can be formulated in different imaging frameworks, depending on the type of data used and image optimization criteria. Examples of imaging frameworks correspond to zero-offset migration (designed for imaging based on focusing properties of the image), survey-sinking migration (designed for imaging based on moveout analysis using narrow-azimuth data) and shot-record migration (also designed for imaging based on moveout analysis, but using wide-azimuth data). The wave-equation MVA operators formulated for the various imaging frameworks are similar because they share common elements derived from linearizations of the single square-root equation. Such operators represent the core of iterative velocity estimation based on diffraction focusing or semblance analysis, and their applicability in practice requires efficient and accurate implementation. This tutorial concentrates strictly on the numeric implementation of those operators and not on their use for iterative migration velocity analysis.
Interferometric imaging condition for wave-equation migration [pdf 6.5M]
Paul Sava and Oleg Poliannikov
The fidelity of depth seismic imaging depends on the accuracy of the velocity models used for wavefield reconstruction. Models can be decomposed in two components corresponding to large scale and small scale variations. In practice, the large scale velocity model component can be estimated with high accuracy using repeated migration/tomography cycles, but the small scale component cannot. When the Earth has significant small-scale velocity components, wavefield reconstruction does not completely describe the recorded data and migrated images are perturbed by artifacts. There are two possible ways to address this problem: improve wavefield reconstruction by estimating more accurate velocity models and image using conventional techniques (e.g. wavefield cross-correlation), or reconstruct wavefields with conventional methods using the known background velocity model, but improve the imaging condition to alleviate the artifacts caused by the imprecise reconstruction, which is what we suggest in this paper. We describe the unknown component of the velocity model as a random function with local spatial correlations. Imaging data perturbed by such random variations is characterized by statistical instability, i.e. various wavefield components image at wrong locations that depend on the actual realization of the random model. Statistical stability can be achieved by pre-processing the reconstructed wavefields prior to the imaging condition. We employ Wigner distribution functions to attenuate the random noise present in the reconstructed wavefields, parametrized as a function of image coordinates. Wavefield filtering using Wigner distribution functions and conventional imaging can be lumped-together into a new form of imaging condition which we call an ``interferometric imaging condition'' due to its similarity to concepts from recent work on interferometry. The interferometric imaging condition can be formulated both for zero-offset and for multi-offset data, leading to robust and efficient imaging procedures that are effective in attenuating imaging artifacts due to unknown velocity models.
Isotropic angle-domain elastic reverse-time migration [pdf 1.5M]
Jia Yan and Paul Sava
Multicomponent data are not usually processed with specifically designed procedures, but with procedures analogous to the ones used for single-component data. In isotropic media, the vertical and horizontal components of the data are commonly taken as proxies for the P- and S-wave modes which are imaged independently with acoustic wave equations. This procedure works only if the vertical and horizontal component accurately represent P- and S-wave modes, which is not true in general. Therefore, multicomponent images constructed with this procedure exhibit artifacts caused by the incorrect wave mode separation at the surface. An alternative procedure for elastic imaging uses the full vector fields for wavefield reconstruction and imaging. The wavefields are reconstructed using the multicomponent data as a boundary condition for a numerical solution to the elastic wave equation. The key component for wavefield migration is the imaging condition that evaluates the match between wavefields reconstructed from sources and receivers. For vector wavefields, a simple component-by-component cross-correlation between two wavefields leads to artifacts caused by crosstalk between the unseparated wave modes. An alternative method is to separate elastic wavefields after reconstruction in the subsurface and implement the imaging condition as cross-correlation of pure wave modes instead of the Cartesian components of the displacement wavefield. This approach leads to images that are easier to interpret, since they describe reflectivity of specified wave modes at interfaces of physical properties. As for imaging with acoustic wavefields, the elastic imaging condition can be formulated conventionally (cross-correlation with zero lag in space and time), as well as extended to non-zero space and time lags. The elastic images produced by an extended imaging condition can be used for angle decomposition of primary (PP or SS) and converted (PS or SP) reflectivity. Angle gathers constructed with this procedure have applications for migration velocity analysis and amplitude versus angle analysis.

2009

Elastic wave-mode separation for TTI media [pdf 3.1M]
Jia Yan and Paul Sava
Seismic waves propagate through the earth as a superposition of different wave-modes. Seismic imaging in areas characterized by complex geology requires techniques based on accurate reconstruction of the seismic wavefields. A crucial component of the methods in this category, collectively known as wave-equation migration, is the imaging condition which extracts information about the discontinuities of physical properties from the reconstructed wavefields at every location in space. Conventional acoustic migration techniques image a scalar wavefield representing the P wave-mode, in contrast with elastic migration techniques which image a vector wavefield representing both the P and S wave-modes. For elastic imaging, it is desirable that the reconstructed vector fields are decomposed in pure wave-modes, such that the imaging condition produces interpretable images, characterizing for example PP or PS reflectivity. In anisotropic media, wave-mode separation can be achieved by projection of the reconstructed vector fields on the polarization vectors characterizing various wave modes. For heterogeneous media, the polarization directions change with position, therefore wave-mode separation needs to be implemented using space-domain filters. For transversely isotropic media with a tilted symmetry axis (TTI), the polarization vectors depend on the elastic material parameters, including the tilt angles. Using these parameters, I separate the wave-modes by constructing nine filters corresponding to the nine Cartesian components of the three polarization directions at every grid point. Since the S polarization vectors in TI media are not defined in the singular directions, e.g. along the symmetry axes, I construct these vectors by exploiting the orthogonality between the SV and SH polarization vectors, as well as their orthogonality with the P polarization vector. This procedure allows one to separate S wave-modes which are only kinematically correct. Realistic synthetic examples show that this wave-mode separation is effective for both 2D and 3D models with high heterogeneity and strong anisotropy.
Elastic wave-mode separation for VTI media [pdf 3.8M]
Jia Yan and Paul Sava
Elastic wave propagation in anisotropic media is well represented by elastic wave equations. Modeling based on elastic wave equations characterizes both kinematics and dynamics correctly. However, because P and S modes are both propagated using elastic wave equations, there is a need to separate P and S modes to obtain clean elastic images. The separation of wave modes to P and S from isotropic elastic wavefields is typically done using Helmholtz decomposition. However, Helmholtz decomposition using conventional divergence and curl operators in anisotropic media does not give satisfactory results and leaves the different wave modes only partially separated. The separation of anisotropic wavefields requires the use of more sophisticated operators which depend on local material parameters. Anisotropic wavefield separation operators are constructed using the polarization vectors evaluated by solving the Christoffel equation at each point of the medium. These polarization vectors can be represented in the space domain as localized filtering operators, which resemble conventional derivative operators. The spatially-variable ``pseudo'' derivative operators perform well in heterogeneous VTI media even at places of rapid velocity/density variation. Synthetic results indicate that the operators can be used to separate wavefields for VTI media with an arbitrary degree of anisotropy.

2011

Wide-azimuth angle gathers for wave-equation migration [pdf 1.9M]
Paul Sava and Ioan Vlad
Extended common-image-point-gathers (CIP) contain all the necessary information for decomposition of reflectivity as a function of the reflection and azimuth angles at selected locations in the subsurface. This decomposition operates after the imaging condition applied to wavefields reconstructed by any type of wide-azimuth migration method, e.g. using downward continuation or time reversal. The reflection and azimuth angles are derived from the extended images using analytic relations between the space-lag and time-lag extensions. The transformation amounts to a linear Radon transform applied to the CIPs obtained after the application of the extended imaging condition. If information about the reflector dip is available at the CIP locations, then only two components of the space-lag vectors are required, thus reducing computational cost and increasing the affordability of the method. Applications of this method include the study of subsurface illumination in areas of complex geology where ray-based methods are not usable, and the study of amplitude variation with reflection and azimuth angles if the subsurface subsurface illumination is sufficiently dense. Migration velocity analysis could also be implemented in the angle domain, although an equivalent implementation in the extended domain is cheaper and more effective.
Micro-earthquake monitoring with sparsely-sampled data [pdf 3.2M]
Paul Sava
Micro-seismicity can be used to monitor the migration of fluids during reservoir production and hydro-fracturing operations in brittle formations or for studies of naturally occurring earthquakes in fault zones. Micro-earthquake locations can be inferred using wave-equation imaging under the exploding reflector model, assuming densely sampled data and known velocity. Seismicity is usually monitored with sparse networks of seismic sensors, for example located in boreholes. The sparsity of the sensor network itself degrades the accuracy of the estimated locations, even when the velocity model is accurately known. This constraint limits the resolution at which fluid pathways can be inferred. Wavefields reconstructed in known velocity using data recorded with sparse arrays can be described as having a random character due to the incomplete interference of wave components. Similarly, wavefields reconstructed in unknown velocity using data recorded with dense arrays can be described as having a random character due to the inconsistent interference of wave components. In both cases, the random fluctuations obstruct focusing that occurs at source locations. This situation can be improved using interferometry in the imaging process. Reverse-time imaging with an interferometric imaging condition attenuates random fluctuations, thus producing crisper images which support the process of robust automatic micro-earthquake location. The similarity of random wavefield fluctuations due to model fluctuations and sparse acquisition are illustrated in this paper with a realistic synthetic example.

CWP -- TABLE OF CONTENTS